The assumption of a global definite causal order, typical of temporal correlations, can be relaxed, leading to the so-called process matrix formalism [1]. There, stronger spatio-temporal correlations are possible than in the causally ordered case. The object of investigation are correlations between classical inputs and outputs, which are computed as
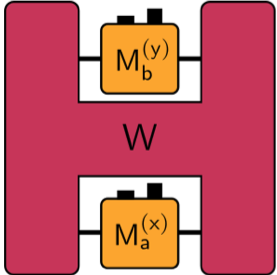
pout|in(ab|xy) = tr[W(Ma(x) ⊗ Mb(y))] ,
where Ma(x), Mb(y) represent the local operations (see Fig.1). The process matrix W contains all spatio-temporal correlations, but does not have to correspond to a causally ordered process and only satisfies weaker constraints. In particular it allows for “superpositions of causal orders” and, in some instances, cannot be separated into causally ordered objects. This means that in a bipartite scenario, when looking purely at the process matrix, or even the correlations pout|in(ab|xy)(results of the experiments) that it produces, it is simply impossible to assign the statements “A went before B” or “B went before A” (or probabilistic mixtures of these two scenarios).
While not a priori prohibited by physical principles, the physicality of causally indefinite process matrices is subject of lively debate. Our research in this field, thus, focuses on two main points: (a) Investigating in what sense and to what extent causally indefinite correlations go beyond what is possible in a causally ordered world; (b) Identifying physical principles that limit and stratify the set of possible process matrices.
[1] O. Oreshkov, F. Costa, and Č. Brukner, "Quantum correlations with no causal order", Nature Communications 3, 1092 (2012).
[2] C. Branciard, M. Araújo, A. Feix, F. Costa and Č. Brukner, “The simplest causal inequalities and their violation”, New J. Phys. 18, 013008 (2016).
[3] M. Araújo, A. Feix, M. Navascués and Č. Brukner, "A purification postulate for quantum mechanics with indefinite causal order", Quantum 1, 10 (2017).
[4] N. Miklin, A. A. Abbott, C. Branciard, R. Chaves, and C. Budroni, The entropic approach to causal correlations, New J. Phys. 19 113041 (2017).
[5] Y. Guryanova, R. Silva, A. J. Short, P. Skrzypczyk, N. Brunner, and S. Popescu, "Exploring the limits of no backwards in time signalling", Quantum 3, 211 (2019).
