Caslav Brukner
- Scientific & Deputy Executive Director
- Group Leader
- +43 (1) 4277 - 72582
- Caslav.Brukner(at)oeaw.ac.at
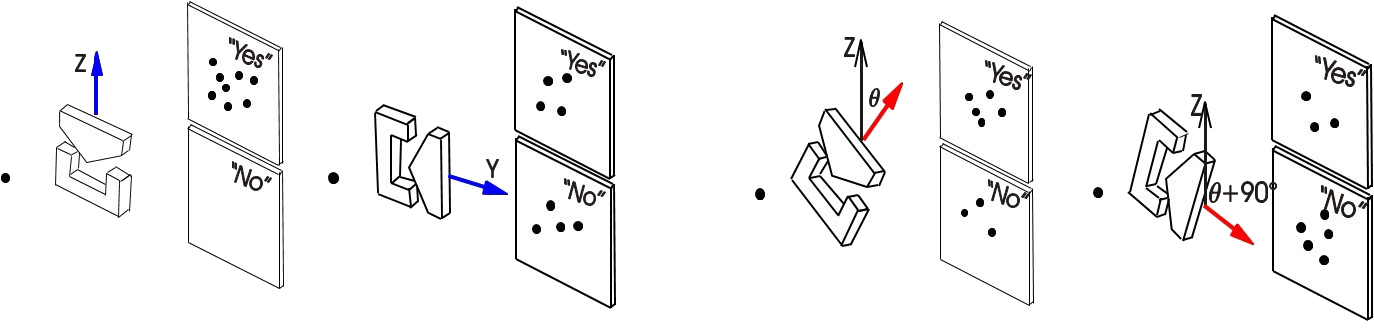
Motivated by Wheeler’s idea of “it from bit” – the idea that information is the most fundamental, basic entity – we formulated one of the first proposals for an “information-theoretic” approach to quantum theory in 1999 [1,2]. The basic idea is that every quantum system is associated with a finite amount of information. Alternatively, one can say that the most elementary system carries one bit of information, as represented by the truth value of an elementary proposition. An example of a one-bit proposition is: “The spin is up along z-direction”, which describes a Stern-Gerlach experiment in abstract information-theoretic language.
Many fundamental features of quantum systems can be understood as consequences of the constraints that limited information content imposes on the systems [1,2]. Due to the limited information content an increase in the knowledge of one of the observables is at the expense of the corresponding decrease of the knowledge in others, complementary observables. Entanglement arises from the possibility that the information in a composite system may reside more in the correlations between systems than in the individual systems themselves. Finally, the information content of a system remains constant at all times giving rise to unitarity of the dynamics in quantum theory.
These considerations led to an information-theoretic reconstruction of quantum theory [3] (See also “Reconstruction of Quantum Theory”).
[1] Č. Brukner and A. Zeilinger, Operationally Invariant Information in Quantum Measurements, Phys. Rev. Lett. 83, 3354 (1999).
[2] Č. Brukner and A. Zeilinger, Information and Fundamental Elements of the Structure of Quantum Theory, in “Time, Quantum, Information”, Ed. L. Castell and O Ischebeck (Springer, 2003). Preprint at quant-ph/0212084.
[3] Č. Brukner and A. Zeilinger, Information Invariance and Quantum Probabilities, Found. Phys. 39, 677 (2009).
H. C. von Bayer, In the beginning was the bit, Feature of New Scientist (27 March 2004)